Table of Contents
Doubling time (td) indicates how long the present population will take to double from its present or initial size. It is a measure of how rapidly a population grows.
Doubling time related to the equation of exponential growth
The td for an exponential function N0ert is the time td (doubling time) that gives a population of 2 X N0. Thus, we can get the doubling time by solving the equation:
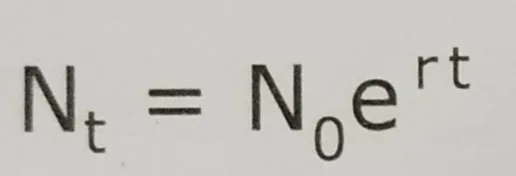

Deduction of the equation:
Nt = N0ert
Nt= population density at time t
N0= population density at time 0
r= intrinsic rate of natural increase
t= time interval
e= the base of the natural logarithm (constant about 2.7)
The term e to the power r is the factor by which the population increases during each time unit.
Nt = N0ert
or, 2 X N0 = N0ertd
or, ertd = 2
[This equation indicates that rtd is the power of e that gives 2.]
or, rtd = ln 2
or, td = ln 2/r
Final formula for the calculation:
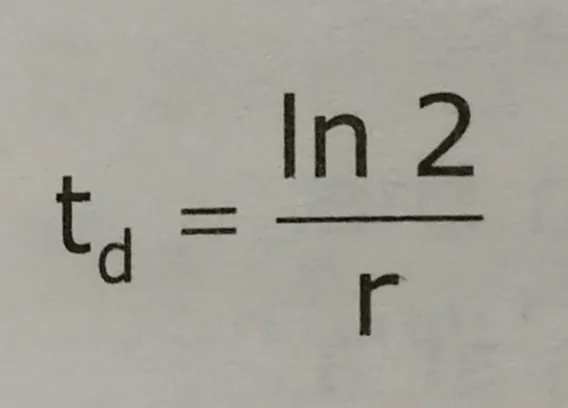
Where, td = Doubling time; r = intrinsic rate of increase
Conclusion:
From the above equation, if the intrinsic rate of increase (r) is easily known, the td of a population can be easily calculated. However, we assume that the population is not affected by its age distribution, and that r (the intrinsic rate of increase) is a constant during this period.
Other related link:
- Population growth- Geometric growth: https://thebiologyislove.com/population-growth-geometric-growth/
- Population growth- Exponential growth: https://thebiologyislove.com/population-growth-exponential-growth/
Facebook link: https://www.facebook.com/share/p/FpUSTdy4VfA9kyu5/?mibextid=oFDknk
Instagram link: https://www.instagram.com/p/C7d9ZVCxNGH/?igsh=enoxNXVkNnVlMjY0
